An icosahedron is a platonic solid. Its faces, like the thetrahedron and the octahedron, are equilateral triangles.

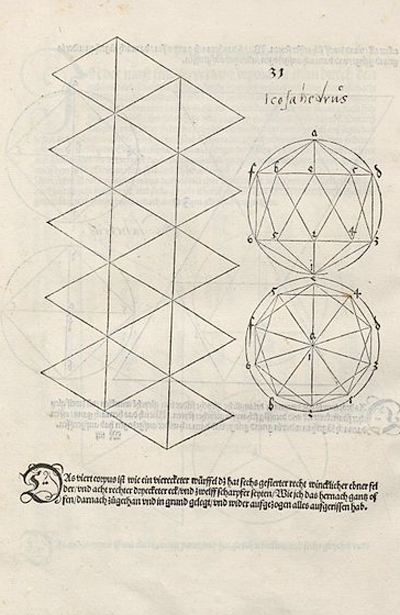
It is very easy to build one using its plane net, as Dürer taught us in his book 'Underweysung der Messung' ('Four Books on Measurement') published in 1525:

Or we can make this beautiful lamp:

In this page we are going to study the relation between the golden ratio and the icosahedron.

The golden rectangle is a beautiful construction also related with some spirals and the dodecahedron.

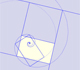
We can put three golden rectangles (in three mutually orthogonal planes) and make a well known construction. These rectangles have 12 vertices. The distance between any pair of neighbouring points is equal to the short side of one golden rectangle. Then, these 12 points coincide with the 12 vertices of an icosahedron.

By symmetry, these twelve triangles are isosceles triangles but, they are equilateral?

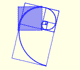
One easy way to convince yourself about that is calculating the height of one of these triangles:

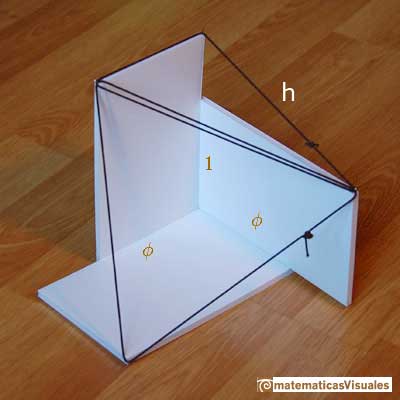
Remember that

Then

And h is the height of a equilateral triangle of side length 2:

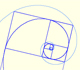
We are going to calculate the volumen of an icosahedron of side length 2:

An icosahedron has twenty equilateral triangles.

The area of one of the twenty equilateral triangles that the icosahedron of side 2 have is:

The volume of an icosahedron of side 1 is one eigth of the volume of an icosahedron of side 2.

The volume of the icosahedron of side 1 is the same as the volume of two and a half pyramids. We need to calculate the height of one of this pyramids: the distance between the center and one triangular face.

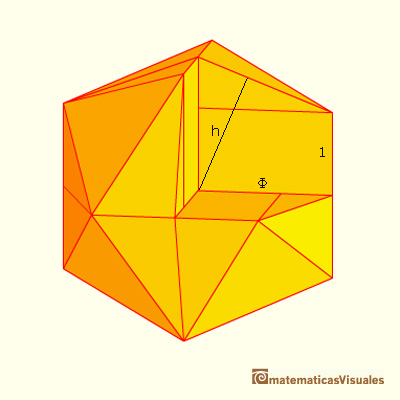
There are two similar triangles and we can write:

Then the volume of one pyramid is:

And the volume of an icosahedron of side 1 is:

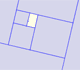
The six vertices of an octahedron lies in three mutually ortogonal squares. We can put inside each square a golden rectangle (for example, using tubes):

The same construction with Zome:

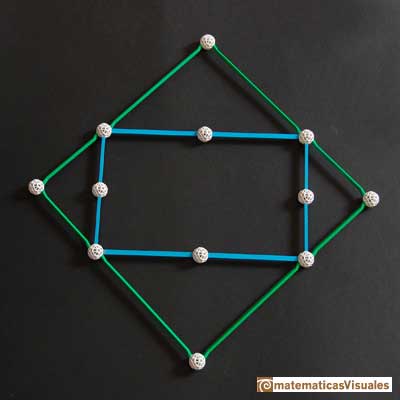
Then, if the twelve edges of an octahedron are divided in the golden ratio (in some order) these vertices are the vertices of an icosahedron.

It is not difficult to calculate the volume of the octahedron and the volume of the six bipyramids and get the volume of the icosahadron.

Icosahedron and octahedron, one inside each other, with Zome: .

An icosahedron, an octahedron and a tetrahedron, one inside each other, made using using tubes:

MORE LINKS

Resources: The golden rectangle and the icosahedron

With three golden rectangles you can build an icosahedron.

Pythagoras Theorem: Euclid's demonstration

Demonstration of Pythagoras Theorem inspired in Euclid.

The Diagonal of a Regular Pentagon and the Golden Ratio

The diagonal of a regular pentagon are in golden ratio to its sides and the point of intersection of two diagonals of a regular pentagon are said to divide each other in the golden ratio or 'in extreme and mean ratio'.

Drawing a regular pentagon with ruler and compass

You can draw a regular pentagon given one of its sides constructing the golden ratio with ruler and compass.

The golden rectangle

A golden rectangle is made of an square and another golden rectangle.

The golden spiral

The golden spiral is a good approximation of an equiangular spiral.

The golden rectangle and two equiangular spirals

Two equiangular spirals contains all vertices of golden rectangles.

The golden rectangle and the dilative rotation

A golden rectangle is made of an square an another golden rectangle. These rectangles are related through an dilative rotation.

Plane developments of geometric bodies: Dodecahedron

The first drawing of a plane net of a regular dodecahedron was published by Dürer in his book 'Underweysung der Messung' ('Four Books of Measurement'), published in 1525 .

Leonardo da Vinci: Drawing of a dodecahedron made to Luca Pacioli's De divina proportione.

Leonardo da Vinci made several drawings of polyhedra for Luca Pacioli's book 'De divina proportione'. Here we can see an adaptation of the dodecahedron.

Volume of a regular dodecahedron

One eighth of a regular dodecahedon of edge 2 has the same volume as a dodecahedron of edge 1.

The volume of the tetrahedron

The volume of a tetrahedron is one third of the prism that contains it.

Plane developments of geometric bodies: Tetrahedron

The first drawing of a plane net of a regular tetrahedron was published by Dürer in his book 'Underweysung der Messung' ('Four Books of Measurement'), published in 1525 .

Volume of an octahedron

The volume of an octahedron is four times the volume of a tetrahedron. It is easy to calculate and then we can get the volume of a tetrahedron.

Dilation and rotation in an equiangular spiral

Two transformations of an equiangular spiral with the same general efect.

Equiangular spiral

In an equiangular spiral the angle between the position vector and the tangent is constant.