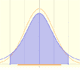
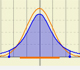
In some cases, a Binomial Distribution can be approximated by a Normal Distribution (with the same mean and variance).

If X is a random variable with a Binomial Distribution we can write:

Then, if Y is a random variable with a Normal Distribution with the same mean and variance:

We can use two approximations. First, we can use the normal mass function: (in the applet this is the orange line)

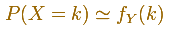
A second approach is better and more practical if we use the tables of a normal distribution. Now we use a continuity correction, for example:

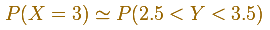
In the applet, this approximation is the red line.

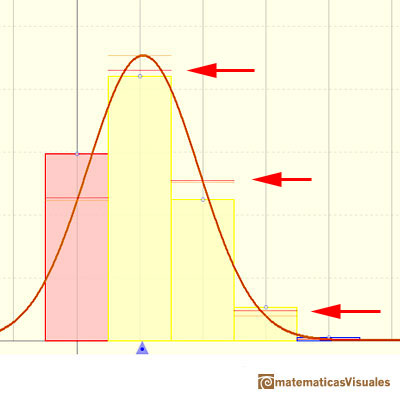
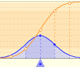
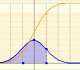
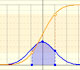
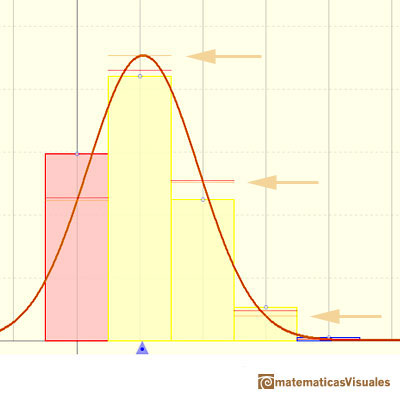
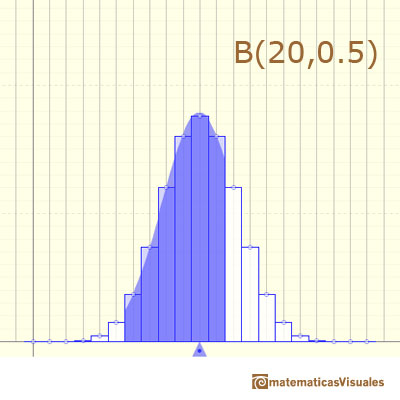
In the applet, when the absolute error of this normal approximation is less than 0.005 we draw a blue rectangle. If the approximation is between 0.005 and 0.05 we draw a yellow rectangle and if the approximation is less accurate the rectangle is red.

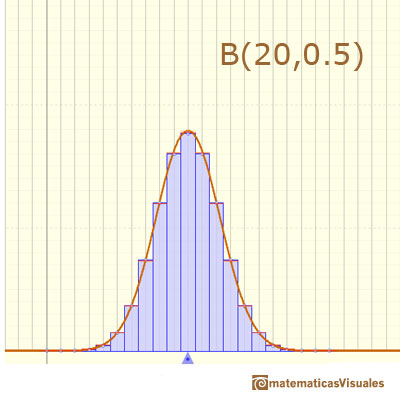
Here we can see a case where the approximation seems accurate:

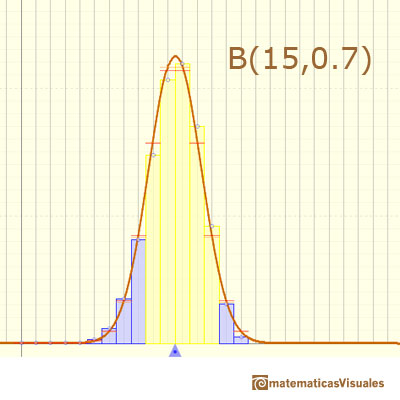
This approximation seems no so good:

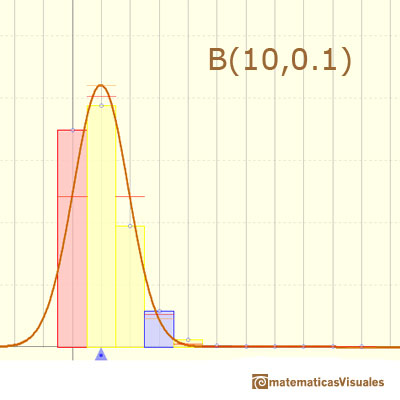
In the next case the approximation is not accurate:

If n is large enough and p is "near" 0.5, then the skew of the distribution is not too great. In this case the normal distribution gives an excellent approximation. Because the normal approximation is not accurate for small values of n, there are several rules of thumb. For example, one is that we can use the normal approximation only if

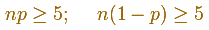
The approximation generally improves as n increases and is better when p is not near 0 or 1.

Here we can see a typical example of the use of the normal tables to calculate probabilities in a Binomial distribution (using the correction for continuity adjustement)

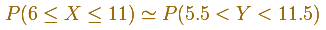
With large n, exact calculations of a Binomial Distribution can be very time consuming. But using a table with the Normal Distribution is easy. Historically, it was the first use of the normal distribution, introduced by Abraham de Moivre.

MORE LINKS

Normal Distributions: One, two and three standard deviations

One important property of normal distributions is that if we consider intervals centered on the mean and a certain extent proportional to the standard deviation, the probability of these intervals is constant regardless of the mean and standard deviation of the normal distribution considered.

Normal Distributions: Probability of Symmetric Intervals

Calculating probabilities of symmetric intervals around the mean of a normal distribution.

Normal Distributions: (Cumulative) Distribution Function

The (cumulative) distribution function of a random variable X, evaluated at x, is the probability that X will take a value less than or equal to x. In this page we study the Normal Distribution.

Poisson distribution

Poisson distribution is discrete (like the binomial) because the values that can take the random variable are natural numbers, although in the Poisson distribution all the possible cases are theoretically infinite.

Student's t-distributions

Student's t-distributions were studied by William Gosset(1876-1937) when working with small samples.

Calculating probabilities in t Student distributions (Spanish)