|
|
|
Septuaginta (adapted from Leonardo da Vinci)
This video is an adaptation of the drawing that Leonardo da Vinci made of a polyhedron with 72 faces like a sphere (septuaginta duarum basium vacuus) for Luca Pacioli's book 'De Divina Proportione'.
Pacioli wrote about this polyhedron (Spanish translation):
"Entre estos cuerpos conviene colocar, Excelso Duque, el llamado cuerpo de setenta y dos bases, del que da una plena descripción
nuestro filósofo megarense en la decimocuarta de su duodécimo. Dicho cuerpo, aunque tiene sus bases planas laterales y angulares y
deformes, no se puede decir que dependa o derive de alguno de los cuerpos regulares, sino que se forma y se crea, según lo que en
dicho lugar demuestra nuestro filósofo, mediante la figura dodecagonal, es decir, de doce lados iguales. Cuarenta y dos de sus bases
son cuadrangulares, no equiláteras ni equiángulas, y tienen sus dos lados opuestos dirigidos hacia uno y otro polo o cono e iguales
entre sí; sus otras veinticuatro bases son triangulares, igualmente no equiláteras; doce de ellas están en torno a uno de los conos
y doce en torno al otro, y cada una de ellas tiene dos lados iguales, los que tienden hacia el punto del polo inferior y superior. (...)
Este cuerpo de setenta y dos bases es muy frecuentemente empleado por los arquitectos en sus disposiciones de edificios, por ser
forma bastante útil, sobre todo cuando hay que hacer tribunas y otras bóvedas o cielos."
('La divina proporción' de Luca Pacioli, page 99, Spanish translation by Juan Calatrava, Editorial Akal, 4th edition, 2008)
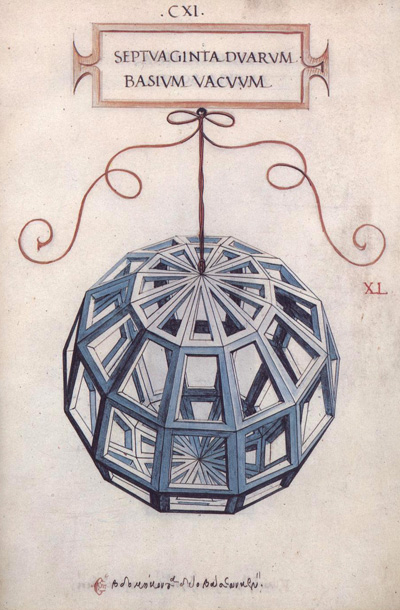
Leonardo da Vinci's drawing of a polyhedron with 72 faces like a sphere (septuaginta duarum basium vacuus) for Luca Pacioli's book 'De divina proportione'. (There is an Spanish version, 'La divina proporción' Editorial Akal. Image used with permission of Editorial Akal).
Leonardo da Vinci's drawing of a polyhedron with 72 faces like a sphere (septuaginta duarum basium solidus) for Luca Pacioli's book 'De divina proportione'. (There is an Spanish version, 'La divina proporción' Editorial Akal. Image used with permission of Editorial Akal).
Polyhedra of this kind are useful in Architecture and art. One example in Madrid (Spain):
If you play with the interactive application you can get images like these:
These polyhedra (with some restrictions) are called Campanus'sphere. There were very popular during the Reinaissance.
REFERENCES
Luca Pacioli - La divina proporción - Ediciones Akal, 4th edition, 2004. Spanish edition of 'De divina proportione'. Translation by Juan Calatrava.
Peter R. Cromwell - 'Polyhedra', Cambridge University Press, 1999.
Leonardo da Vinci's Polyhedra and
Fra Giovanni's Intarsia Polyhedra at
George Hart's excellent website about polyhedra.
Fra' Gioavanni`s Intarsias in Verona,
article by H. Thomas and K. Williams (The Mathematical Tourist).
Some images of a beautiful intarsia in the Monastery of El Escorial (Spain).
Leonardo da Vinci's Geometric Sketches Frank J. Swetz's article in MathDl, Loci:Convergence.
Biographies of
Luca Pacioli,
Leonardo da Vinci and
Campanus of Novara
at MacTutor History of Mathematics archive.
NEXT
We study a kind of polyhedra inscribed in a sphere, in particular the Campanus' sphere that was very popular during the Renaissance.
PREVIOUS
Leonardo da Vinci made several drawings of polyhedra for Luca Pacioli's book 'De divina proportione'. Here we can see an adaptation of the rhombicuboctahedron.
MORE LINKS
We want to calculate the surface area of sections of a sphere using the Pythagorean Theorem. We also study the relation with the Geometric Mean and the Right Triangle Altitude Theorem.
Using Cavalieri's Principle we can calculate the volume of a sphere.
Leonardo da Vinci made several drawings of polyhedra for Luca Pacioli's book 'De divina proportione'. Here we can see an adaptation of the octahedron.
Leonardo da Vinci made several drawings of polyhedra for Luca Pacioli's book 'De divina proportione'. Here we can see an adaptation of the truncated tetrahedron.
Leonardo da Vinci made several drawings of polyhedra for Luca Pacioli's book 'De divina proportione'. Here we can see an adaptation of the truncated octahedron.
Leonardo da Vinci made several drawings of polyhedra for Luca Pacioli's book 'De divina proportione'. Here we can see an adaptation of the dodecahedron.
Leonardo da Vinci made several drawings of polyhedra for Luca Pacioli's book 'De divina proportione'. Here we can see an adaptation of the stellated octahedron (stella octangula).
Leonardo da Vinci made several drawings of polyhedra for Luca Pacioli's book 'De divina proportione'. Here we can see an adaptation of the cuboctahedron.
The volume of an octahedron is four times the volume of a tetrahedron. It is easy to calculate and then we can get the volume of a tetrahedron.
A cuboctahedron is an Archimedean solid. It can be seen as made by cutting off the corners of a cube.