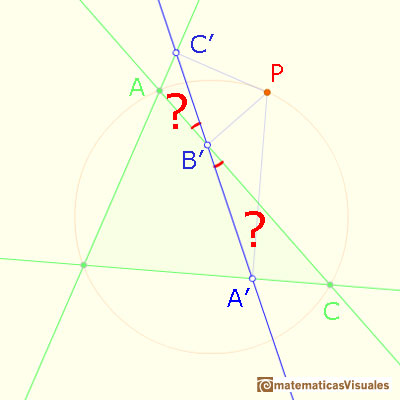
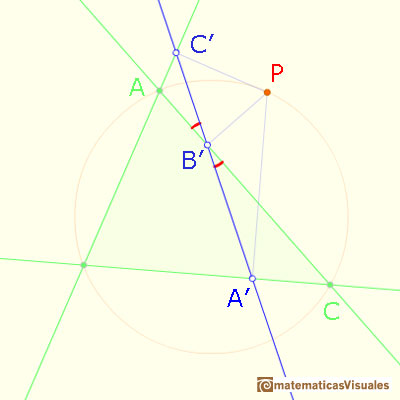
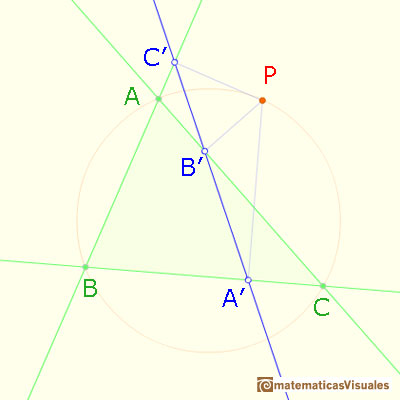
We can start with a triangle and its circunscribed circle. Given a point P on the circumcircle of a triangle, the feet of the perpendiculars from P to the three sides all lie on a straight line (Simson line or Simson-Wallace line)

Wallace-Simson lines

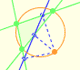
Each point in the circle circunscribed to a triangle give us a line (Wallace-Simson line)

We are going to see this property using this notation:

We have taken P to lie on the arc AC that does not contain B. Other cases can be derived by re-naming A, B, C.

If we can prove that these two angles are equal then points A', B', C' will be collinear.

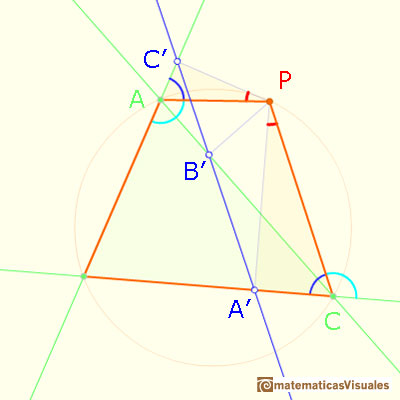
We can use a consequence of a circle property (Euclides, III.21 or III.22) that saids that the opposite angles of every convex cuadrangle inscribed in a circle are together equal to two right angles.

Central and inscribed angles in a circle

Central angle in a circle is twice the angle inscribed in the circle.

Two right triangles are similar, then:

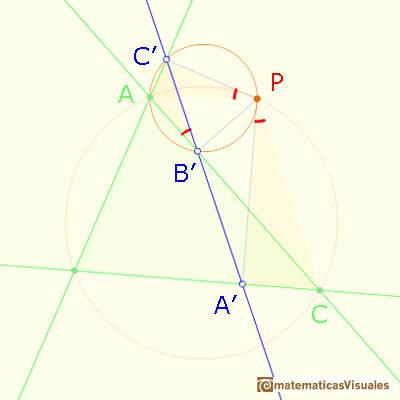
Points A, B', P, C' lies on a circle:

And points B',A',C,P lies on a circle:

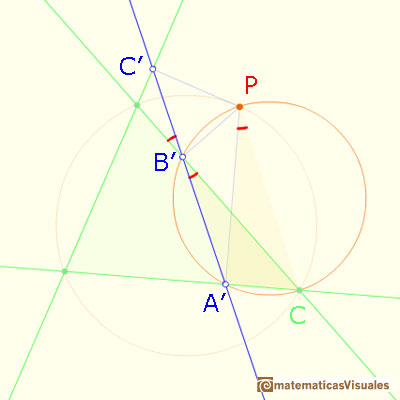
Then points A', B', C' are collinear. This is called Simson Line or Simson-Wallace Line of P.

MORE LINKS

Steiner deltoid is a hypocycloid

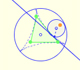
Steiner deltoid is a hypocycloid related with the nine point circle of a triangle.

The deltoid and the Morley triangle

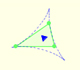
Steiner Deltoid and the Morley triangle are related.

Central and inscribed angles in a circle

Central angle in a circle is twice the angle inscribed in the circle.

Central and inscribed angles in a circle | Mostration | Case I

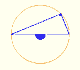
Interactive 'Mostation' of the property of central and inscribed angles in a circle. Case I: When the arc is half a circle the inscribed angle is a right angle.

Central and inscribed angles in a circle | Mostration | Case II

Interactive 'Mostation' of the property of central and inscribed angles in a circle. Case II: When one chord that forms the inscribed angle is a diameter.

Central and inscribed angles in a circle | Mostration | General Case

Interactive 'Mostation' of the property of central and inscribed angles in a circle. The general case is proved.

Drawing fifteen degrees angles

Using a ruler and a compass we can draw fifteen degrees angles. These are basic examples of the central and inscribed in a circle angles property.

Morley Theorem

The three points of intersection of the adjacent trisectors of the angles of any triangle are the vertices of an equilateral triangle (Morley's triangle)

John Conway's proof of Morley's Theorem

Interactive animation about John Conway's beautiful proof of Morley's Theorem