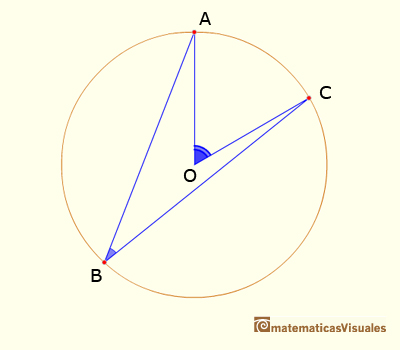
The general case of the Central Angle Theorem can be proved using the previous case. To do that we can draw the diameter that pases through the vertex of the inscribed angle. Then, depending on the position of the points, it is enough to add or to subtract some angles.

Central and inscribed angles in a circle

Central angle in a circle is twice the angle inscribed in the circle.

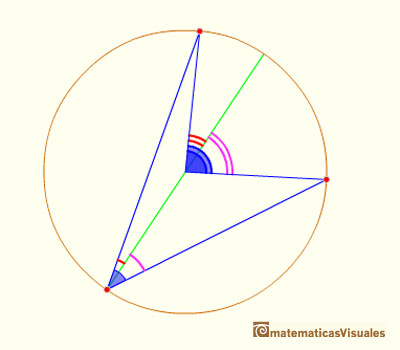
In this position we have to add two central angles (and we use Case II):

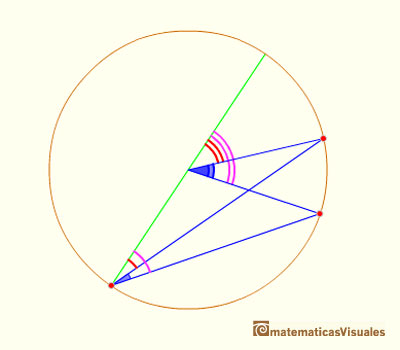
In this position we have to substract two central angles (and we use Case II):

With Case I, Case II and this General Case we have finished the demostration of the Central Angle Theorem.

MORE LINKS

Central and inscribed angles in a circle

Central angle in a circle is twice the angle inscribed in the circle.

Drawing fifteen degrees angles

Using a ruler and a compass we can draw fifteen degrees angles. These are basic examples of the central and inscribed in a circle angles property.

Pythagoras Theorem: Euclid's demonstration

Demonstration of Pythagoras Theorem inspired in Euclid.

Wallace-Simson lines

Each point in the circle circunscribed to a triangle give us a line (Wallace-Simson line)

Wallace-Simson lines | Demonstration

Interactive demonstration of the Wallace-Simson line.

Drawing a regular pentagon with ruler and compass

You can draw a regular pentagon given one of its sides constructing the golden ratio with ruler and compass.

Durer's approximation of a Regular Pentagon

In his book 'Underweysung der Messung' Durer draw a non-regular pentagon with ruler and a fixed compass. It is a simple construction and a very good approximation of a regular pentagon.

The Diagonal of a Regular Pentagon and the Golden Ratio

The diagonal of a regular pentagon are in golden ratio to its sides and the point of intersection of two diagonals of a regular pentagon are said to divide each other in the golden ratio or 'in extreme and mean ratio'.